Spinodal Decomposition
We investigate the dynamics of first-order phase transitions of liquid-vapor systems via spinodal decomposition. The simulation method is based on the common Smoothed Particle Hydrodynamics (SPH) [d4] in its modern formulation with several extensions comprising thermal conduction [d3], the van der Waals equation of state [d2] and a newly developed scaling thermostat. Spinodal decompostion occurs after a sudden quench to the completely unstable spinodal region. The system instantaneously initiates to separate, where the so-called "homphase fluctuations" of the density arise [d1]. We further study the late stage growth behavior of the separated phases, that can be expressed in terms of a scaling law. There, we are mainly interested in the late stage of domain growth, where the role of inertial hydrodynamics is dominating.
Gallery
The videos in the gallery illustrate some effects of the thermostat.
-
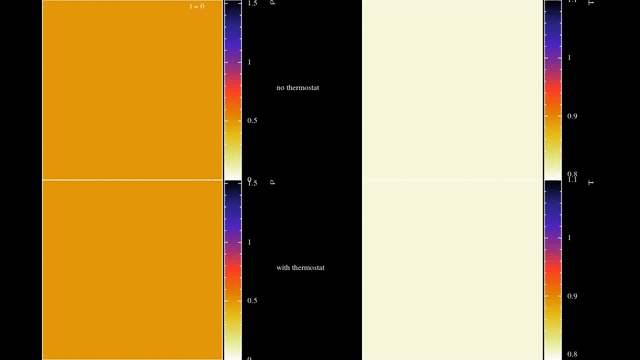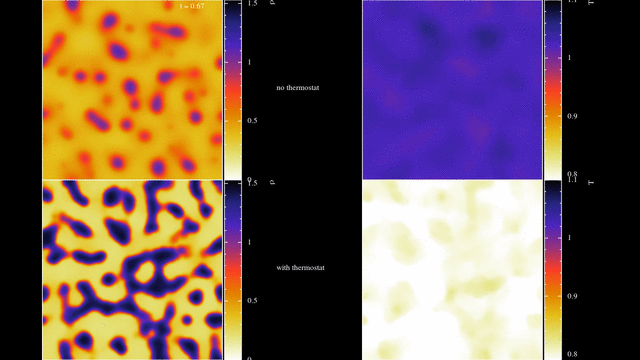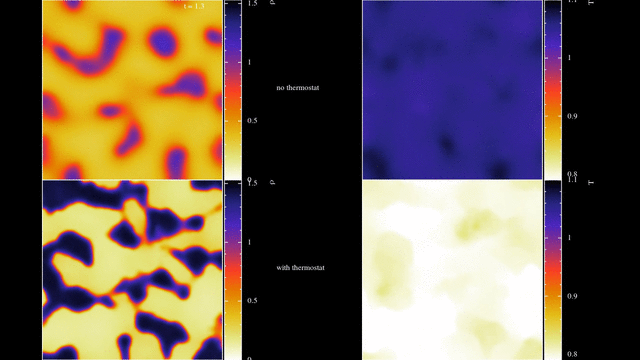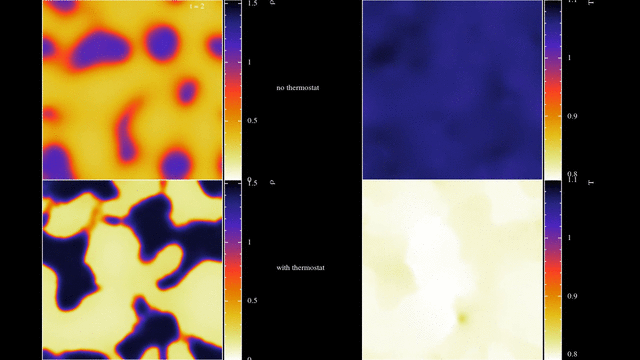
Early stage: Initial growth of "homophase fluctuations". Comparison of densities (left) and temperatures (right) between pure thermal (no thermostat) (top) and with applied thermostat (bottom) simulations is shown. -
Late stage: Domain growth and coarsening. Comparison of the densities between pure thermal (top) and with applied thermostat (bottom) is shown. -

Simulation example at T=0.9. Three dimensional visualization. Color coding represents the density values. -

Simulation example at T=0.9. Three dimensional isosurface rendering of a nucleation process of a thermal conducting and viscous fluid.
b) Old publications
c) Completed work
- M. Nitsch, master thesis: Simulation von Modellkolloiden unter Berücksichtigung der Hydrodynamik durch Modifikation des smoothed particle hydrodynamic Verfahrens (2016).
d) References
- K. Binder, Rep. Prog. Phys. 50, 783 (1987).
- S. Nugent and H. A. Posch, Phys. Rev. E 62, 4968 (2000)
- V. Springel, Annu. Rev. Astron. Astrophys. 48, 391 (2010)
- D. J. Price, J. Comput. Phys. 231, 759 (2012)
